Next: Tensor Einstein Equations
Up: Einstein Equations
Previous: Scalar Einstein Equations
The vector metric source evolution is similarly constructed from
a ``Poisson'' equation: in the generalized Newtonian gauge
| 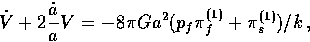 |
(44) |
and for the synchronous gauge,
| 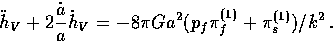 |
(45) |
Likewise momentum conservation implies the Euler equation
| 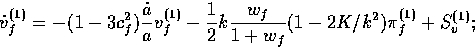 |
(46) |
where recall 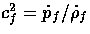 is the sound speed and the
gravitational sources are
is the sound speed and the
gravitational sources are
| 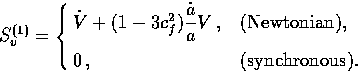 |
(47) |
The seed Euler equation is given by
| 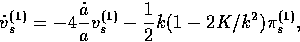 |
(48) |
Again, the first of these equations remains true for each fluid
individually save for momentum exchange terms. The baryon Euler equation has
an additional term in the source of the same form as
Eq. (A12) with 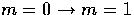 .
.
Wayne Hu
9/9/1997