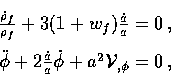Next: Gauge Transformations
Up: Einstein Equations
Previous: Einstein Equations
The Einstein equations
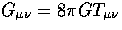 express the metric evolution
in terms of the matter sources. The background evolution equations
are
express the metric evolution
in terms of the matter sources. The background evolution equations
are
for the fluid and scalar field components respectively and
| 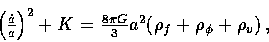 |
(36) |
where 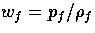 and
and
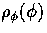 was given in Eq. (17)
and
was given in Eq. (17)
and 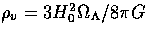 is the vacuum energy.
is the vacuum energy.
Wayne Hu
9/9/1997
![]() express the metric evolution
in terms of the matter sources. The background evolution equations
are
express the metric evolution
in terms of the matter sources. The background evolution equations
are