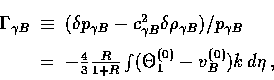Next: Dissipation
Up: Photon-Baryon Fluid
Previous: Viscosity and Polarization
Differences in the bulk velocities of the photons and baryons
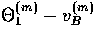 also represent imperfections in the
fluid that lead to entropy generation and heat conduction.
The baryon Euler equations (67) and (71)
give
which may be iterated to the desired order in
also represent imperfections in the
fluid that lead to entropy generation and heat conduction.
The baryon Euler equations (67) and (71)
give
which may be iterated to the desired order in 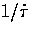 .For scalar fluctuations, this slippage
leads to the generation of non-adiabatic
pressure or entropy fluctuations
as the local number density of baryons to photons changes.
Equivalently, this can be viewed as heat conduction in the fluid.
For vorticity fluctuations, these processes do not occur since there
are no density, pressure, or temperature differentials in the fluid.
.For scalar fluctuations, this slippage
leads to the generation of non-adiabatic
pressure or entropy fluctuations
as the local number density of baryons to photons changes.
Equivalently, this can be viewed as heat conduction in the fluid.
For vorticity fluctuations, these processes do not occur since there
are no density, pressure, or temperature differentials in the fluid.
Wayne Hu
9/9/1997
![\begin{displaymath}
\begin{array}
{rcl}\displaystyle{}\Theta_1^{(0)}- v_B^{(0)}&...
...ot V + {\dot a \over a} (v_B^{(1)}- V)
\right] \, ,\end{array}\end{displaymath}](img358.gif)
![\begin{displaymath}
\begin{array}
{rcl}\displaystyle{}\Theta_1^{(0)}- v_B^{(0)}&...
...ot V + {\dot a \over a} (v_B^{(1)}- V)
\right] \, ,\end{array}\end{displaymath}](img358.gif)