A spatial temperature fluctuation on the last scattering surface appears to us as an anisotropy on the sky. The conversion from physical scale into angular scale depends on the curvature of the universe and the distance to the last scattering surface. The former can alternately be thought of as gravitational lensing from the background curvature rather than curvature fluctuation. Consider first the case of positive curvature:
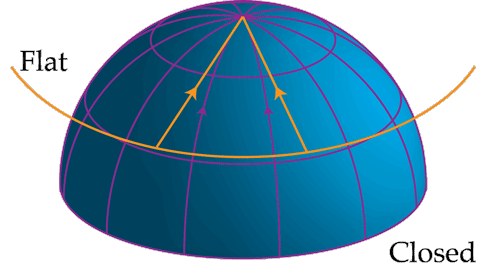
Figure: Closed Universe
Photons free stream to the observer on geodesics analogous to lines of longitude to the pole. Thus the same angular scale represents a smaller physical scale in a closed universe.
The opposite effect is true of open universes:
Figure: Open Universe
Acoustic features appear at harmonics of a fixed physical scale at last scattering, the sound horizon. Measurement of the angular scale that such features subtend on the sky can thus measure the curvature of the universe through an angular size distance test.
Decreasing the distance to the last scattering surface decreases the physical scale associated with a given angular scale. We shall see that sources in the foreground of last scattering such as the ISW effect are affected by this property. However for effects that arise purely from the last scattering surface, such as the acoustic features, the presence of curvature merely scales the features in angular or multipole l space
Animation:Angular diameter distance scaling with curvature and lambda (Omega_K=1-Omega_0-Omega_Lambda, fixed Omega_0h^2 and Omega_Bh^2) PS Figure: Examples from Hu & White (1996a)
With the matter-radiation and baryon-photon ratios fixed, acoustic features just scale with the projection or angular size distance. Geodesic deviation brings the features to higher multipoles (smaller angles) in an open universe. In a cosmological constant (Lambda) universe the rapid expansion causes a decrease in the comoving distance to last scattering bringing the features to slightly larger angles. The degeneracy between these two effects is broken at larger angles by the late ISW effect.
The spacing between the peaks provides the most robust test of the curvature. In models where the variation in the gravitational potential is slow compared with the natural frequency of the oscillator, the natural period of the oscillation sets the separation betwen the peaks (see Hu & White 1996a for comparison between concrete models).
Unfortunately uncertainties in the Hubble constant h and to a lesser extent the baryon-photon ratio muddy the situation a bit.
Figure: Peak Separation and Damping Scale vs. &Omega0 from Hu & White (1996a)
Here we have taken extremely conservative uncertainties in the baryon content and realistic uncertainties in the Hubble constant. Note that since we here allow the matter content &Omega0h2 to vary with &Omega0 in the Lambda universe, the scaling of the peaks does not take on the simple form described above.
The damping scale also provides an angular size distance test for the curvature. Because it is sensitive to the Compton mean free path at last scattering, uncertainties in the baryon content introduce a larger uncertainty in pinning down the curvature:
Of course, by combining these scales one can build a measurement of the curvature that is robust to changes in the other cosmological parameters.